
This document covers the SPICELIB routines that deal with rotations and Representations of rotation transformations: matrices, quaternions,
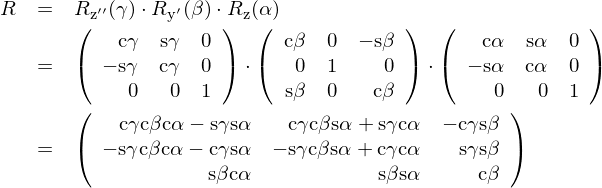

The SPICE rotation routines manipulate and convert between different
Rotation Abstract Introduction Using this document References Notation SPICELIB Routines Categories of routines Euler angle routines Constructing a matrix from Euler angles Finding Euler angles that represent a matrix Programming hazards Working with RA, Dec and Twist Quaternions Finding a quaternion that represents a matrix Finding the matrix represented by a quaternion Q2M and M2Q are approximate inverses of each other Multiplying quaternions Obtaining angular velocity from quaternions Rotating vectors and matrices A word of warning Rotating a vector about a coordinate axis Rotating a matrix about a coordinate axis Rotating a vector about an arbitrary axis Rotation axis and angle Constructing a matrix from a rotation axis and angle Finding the axis and angle of a rotation matrix AXISAR and RAXISA are approximate inverses Using RAXISA and AXISAR Constructing a coordinate axis rotation matrix Rotation derivatives Differentiating rotations State transformations Validating a rotation matrix Tutorial introduction to rotations A comment of the heuristic variety Definition of ``rotation'' Definition 1 Definition 2 Definition 3 Uses of the definitions Definition of ``rotation'' and ``orthogonal'' matrix Rotations preserve inner products Inverses of rotation matrices Composition of rotations Coordinate transformations Rotation of vectors in the plane A canonical representation for rotations Rotation axis and angle Time-dependent coordinate transformations Euler angles Quaternions Quaternion arithmetic Definitions Basic properties of multiplication Deducing the multiplication formula Composing rotations using quaternions Mathematical road map Rotation of a vector about an axis Formation of a rotation matrix from axis and angle Finding the axis and angle of a rotation matrix Formation of a rotation matrix from a quaternion Equivalence of rotation definitions An algebraic approach A geometric approach Quaternion multiplication Assertion 1 Assertion 2 Recovery of Euler angles from a rotation matrix Euler angle recovery: a-b-a case Euler angle recovery: a-b-c case Appendix A: Document Revision History MaNovemJanuFebruDecemApril 26, 1999


 0 kommentar(er)
0 kommentar(er)
